MCC PHS 142 M01 Astronomy Homework Ch.16-17
Adj Prof Astronomy: Sam Wormley <sam.wormley@gmail.com>
Web: edu-observatory.org
Background Material
Textbook - Read Chapters 16-17
Textbook - http://highered.mcgraw-hill.com/sites/0073512184/student_view0/chapter16/
Textbook - http://highered.mcgraw-hill.com/sites/0073512184/student_view0/chapter17/
(take the Multiple Choice Quiz for for each chapter)
Web - http://www.sjaa.net/eph/0612/Cannon.pdf
Web - http://umbra.nascom.nasa.gov/images/latest.html
Web - http://edu-observatory.org/eo/sun.html
Web - http://edu-observatory.org/eo/aurora.html
Web - http://edu-observatory.org/weather/weather_ia.html
Web - http://www.phys.hawaii.edu/~jgl/nuastron.html
Web - http://xxx.lanl.gov/abs/astro-ph/9805135
Web - http://xxx.lanl.gov/pdf/astro-ph/9805135
Web - http://science.nasa.gov/headlines/y2001/ast15feb_1.htm?list125479
Web - http://www.sec.noaa.gov/
Web - http://antwrp.gsfc.nasa.gov/apod/archivepix.html
The Sun - Our Star
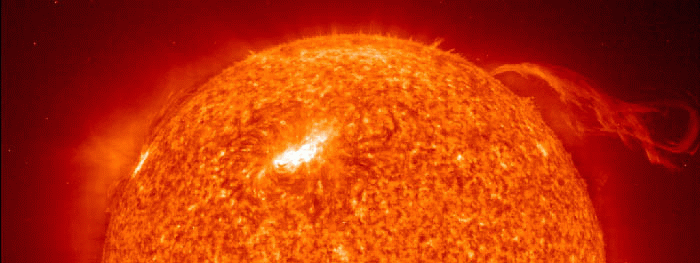The Sun is an ordinary G2 star, one of more than 100 billion stars in
our galaxy.
mass: 1.989e30 kg
temperature: 5800 K (surface), 15,600,000 K (core)
The Sun is by far the largest object in the solar system. It contains
more than 99.8% of the total mass of the Solar System (Jupiter contains
most of the rest).
The Sun is personified in many mythologies: the Greeks called it Helios
and the Romans called it Sol.
The Sun is, at present, about 75% hydrogen and 25% helium by mass
(92.1% hydrogen and 7.8% helium by number of atoms); everything else
("metals") amounts to only 0.1%. This changes slowly over time as the
Sun converts hydrogen to helium in its core.
The outer layers of the Sun exhibit differential rotation: at the
equator the surface rotates once every 25.4 days; near the poles it's
as much as 36 days. This odd behavior is due to the fact that the Sun
is not a solid body like the Earth. Similar effects are seen in the gas
planets. The differential rotation extends considerably down into the
interior of the Sun but the core of the Sun rotates as a solid body.
Conditions at the Sun's core (approximately the inner 25% of its
radius) are extreme. The temperature is 15.6 million Kelvin and the
pressure is 250 billion atmospheres. At the center of the core the
Sun's density is more than 150 times that of water.
The Sun's energy output (3.86e33 ergs/second or 386 billion billion
megawatts) is produced by nuclear fusion reactions. Each second about
700,000,000 tons of hydrogen are converted to about 695,000,000 tons of
helium and 5,000,000 tons (=3.86e33 ergs) of energy in the form of
gamma rays. As it travels out toward the surface, the energy is
continuously absorbed and re-emitted at lower and lower temperatures so
that by the time it reaches the surface, it is primarily visible light.
For the last 20% of the way to the surface the energy is carried more
by convection than by radiation.
Brightness and Color
There are two basic things we can measure from stars, how bright they
are, and what color they are (their color spectra). It is these two
properties that tell us what kind of a star we are looking at. With
successive measurements we can tell if a star is variable, if it has
any measurable parallax and if it has a proper motion.
The Pleiades, an Open Star Cluster
The Pleiades star cluster, often called the "Seven Sisters", is
undoubtedly the most famous galactic star cluster in the heavens, known
and regarded with reverence since remote antiquity. The name is said to
be derived from the Greek word meaning "to sail", from the tradition
that the helical rising of the Pleiades was the sign of the opening
of the navigational season in the Mediterranean world.
In Native American legend the Pleiades are connected with the Mateo
Tepe or Devil's Tower, that curious and wonderfully impressive rock
formation which rises like a colossal petrified tree-stump to a height
of 1300 feet above the plains of northeastern Wyoming. According to the
lore of the Kiowa, the Tower was raised up by the Great Spirit to
protect seven Indian maidens who were pursued by giant bears; the
maidens were afterward placed in the sky as the Pleiades cluster, and
the marks of the bears claws may be seen in the vertical striations on
the sides of the Tower to this day. The Cheyenne had a similar legend.
The brightest members of the Pleiades are accompanied by bright
nebulosity; these are reflection nebulae--dust clouds near the stars
which reflect their light. Eta Tauri or Alcyone, the central star and
brightest member of the Pleiades, is nearly 1000 times more luminous
than the Sun, and probably about 10 times greater in size. The apparent
magnitude is 2.87, spectral type B7e III; the absolute magnitude is
about -2.6. In contrast, the faintest known members are less than
1/100th the solar luminosity. Between these extremes we find the usual
Main Sequence containing all intermediate types and spectra, down to
faint red dwarfs of the 16th magnitude.
When a cloud of pre-stellar material condenses it forms fragments which
contract under their own gravity until the interiors are hot enough for
nuclear reactions to take place. From that moment on the objects are
real stars. Large masses collapse rapidly--in about 100,000 years;
smaller ones less rapidly--in about 10 million years in the case of a
star like the Sun. The energy of a star comes from the inner core of
about 20% of the star's mass, where hydrogen is transformed into helium
with the release of energy which is radiated upwards to the surface of
the star. This is the first phase of a star's life. Theory predicts and
observation confirms that the luminosity of a star increases with its
mass. The luminosity and surface temperature also go hand in hand: the
higher the temperature the greater rate of radiation per unit area.
A graph showing luminosity against surface temperature for stars in the
first phase of their existence is a very smooth curve. It is usually
plotted in the form of absolute magnitude V A against color (B-V) which
is also a measure of its surface temperature, with the most luminous
stars (lowest magnitudes) at the top and the color increasing (redder,
cooler stars) to the right. This curve, called the standard main
sequence, has been built up from observations of stars in nearby
clusters whose distances are known. All stars, from the time of their
birth until the core has used up its hydrogen supply, lie on this
curve.
The color-magnitude diagram of the Pleiades obviously coincides with
the standard main sequence when shifted by the distance modulus of 5.52
mag. The Pleiades cluster lost it most luminous stars because of its
age (65 million years). Only the first 25 stars are used here because
of space, and perhaps, your tolerance. The observed color-magnitude
information for the Pleiades extends several magnitudes fainter than
these and contains about 250 members with measured photoelectric
magnitudes.
Color-magnitude diagrams of star clusters have to be corrected for
interstellar extinction, that is, for the dimming of starlight by dust
in the space between stars. Dust is concentrated in the galactic plane,
and, since open star clusters lie in that plane, all but the nearest of
them are affected by extinction. In the early days of galactic research
it was noticed that cluster distances, estimated from the apparent
magnitudes of member stars, were systematically larger than distances
estimated from the apparent cluster diameters, assuming that all open
clusters more or less the same linear dimensions. The apparent diameter
of a cluster decreases inversely as the distance, while the apparent
brightness of a star decreases inversely as the square of the distance.
The observation that cluster stars appeared dimmer than expected was
one of the pointers to the existence of obscuring material in the
intervening space. The effect of extinction is to make the stars appear
dimmer and redder than they really are. The color-magnitude diagram of
the Pleiades, a nearby cluster, does not suffer from interstellar
extinction.
The method of finding the distance of a cluster by matching the main
sequence is a reliable one, because the use of a large number of stars
smooths out photometric errors in the individual points.
Investigations of the distances of open star clusters have played an
important part in tracing the spiral arms of the Galaxy. Modern
research on color-magnitude diagrams is concerned not only with age and
distance but also with small variations in the evolution of stars in
clusters of different chemical composition.
A remarkable fact about the Pleiades Cluster is that the entire
star-swarm is enveloped in a faint diffuse nebulosity of vast extent
(Tennyson s "silver braid") which appears to shine by reflected light.
This cosmic cloud is elusive visually, but shows much peculiar detail
on long-exposure photographs. The spectrum of this nebulosity is
identical to the spectra of the involved stars, a fact first discovered
by V. M. Slipher at Lowell Observatory in 1912. The brightest portion
of this nebulosity envelops the star Merope, and extends about 20
arcmin to the south; it was first noticed by Professor W. Temple with a
4-inch refractor observing at Venice on October 19th, 1859. He
described it as resembling a faint stain of fog, like the effect of "a
breath on a mirror". Seeing Merope s nebulosity is one of those
benchmark tests that amateurs use to judge the quality of good optics.
To the average eye the Pleiades Cluster appears as a tight knot of 6 or
7 stars, but some observers have recorded 11 or more under excellent
conditions. Miss Agnes Clerke tells us that "Maestlin, the tutor of
Johannes Kepler, perceived 14 stars", and mapped eleven Pleiades
previously to the invention of the telescope; Carrington and Denning
counted fourteen; Miss Airy marked the places of twelve with the naked
eye". Personally, I can consistently see seven of the brightest eight
and often think I can see Pleione next to Atlas in the "handle" of this
"baby dipper". It would be interesting to see how many Pleiades you can
see. You probably need to train your eyes. There are no shortcuts!
The Battle Between Thermal Processes and Gravity
In the Mysteries of Deep Space video we will watch, one astrophysicist
keeps saying that gravity always wins. Gravity tries to collapse the
star, but as long as there is nuclear fuel the star holds itself up
against the pull of gravity. This is a very important concept!
As long as there is nuclear "burning" going on the star holds itself up
against gravity. Our sun has been doing this for about five billion
years, and will do so for another four or five billion years, until it
starts to run out of hydrogen to fuse into helium. For a few more
hundred million years, it will fuse helium into carbon. After that
there is no more nuclear fuel for our sun and gravity will win!
Just about everything that happens in the universe can be considered a
battle between thermal processes and gravity. All matter (and energy)
is attracted to all other matter. Gravity is ceaselessly trying to pull
matter together. Because of the motion of atoms (kinetic theory of
gas), as atoms are crowded together, the temperature increases*, and so
increases the pressure*.
*This is not true for degenerate matter (white dwarfs).
In the case of stars, gravity (sometimes aided by compression from a
supernova shockwave or other perturbation) causes large clouds of gas
(and dust) to collapse under their own weight, forming stars. The
increasing density, and consequently the increasing temperature becomes
so great in the centers of the collapsing gas that thermonuclear
reactions get started. The increased heat given off from the
thermonuclear reactions cause enough outward pressure to halt the
collapse caused by gravity. Observations with the Hubble Space
Telescope show us that star formation is a very violent process
producing jets of ejected material traveling up to a third the speed of
light. The Hubble Space Telescope has also observed many stars with
dark planetary disks a few light years in diameter. These disks will
likely evolve into systems of planets, and other left over material
such as cometary material and asteroids. Eventually the stars come to
equilibrium between heat production and gravity--a balance between the
outward pressure from radiation (heat, light, etc.) and the inward pull
of gravity.
Stars have different evolutionary paths on the Hertzsprung-Russell
(H-R) diagram based on their mass. And they have very different final
fates depending on their mass.
A picture is worth a thousand words.
Look at the diagram on reproduced on the last page. That is the whole
history of our sun plotted in terms of surface brightness as a function
of surface temperature, an H-R diagram. Our sun lasts a little more
than ten billion years with most of its life in thermal equilibrium on
the "Main Sequence".
Homework Problems
Note the answers to the odd (Conceptual Questions, Problems and
Figure-Based Questions) are in the back of your textbook. It is
strongly suggested that you do some of those in every chapter so you
have immediate feedback as how well you are understanding the material.
There are online multiple choice quizzes for each chapter of your
textbook. Goto http://www.mhhe.com/fix then click on
Your book
Student Edition
Choose a chapter
Multiple Choice Quiz
You are expected to do all of your own homework. Statistical patterns
showing copying or collaboration will result in no credit for the
homework assignment for all participants involved. The Code of Academic
Conduct for Iowa Valley Community College District is found in the
Student Handbook.
Physical Science classes require the use of mathematics. If you don't
know algebra, you sould NOT be taking this class. If you need to review,
look at Introduction to Algebra
http://www.math.armstrong.edu/MathTutorial/
WolframAlpha is way faster than a scientific calculator.
http://www.wolframalpha.com
There is little excuse for turning homework in late. You have a whole
week between classes to read the chapters and do the homework. Homework
one week late - half credit. Two or more weeks late - no credit. Do the
homework during the week, not in class! You got homework questions,
email me 24/7. sam.wormley@gmail.com Even if you don't have a homework
question, email me anyway!
Problem 1:
In addition to position, astronomers can record the visual brightness
of a star, V. They can also record the brightness of a star through
filters, in our case a bue filter, B.
Apparent Magnitude, V, of a star, is how bright the star is to our eyes
and instruments. The Absolute Magnitude is defined to be how bright a
star would appear to us if it were at a distance of 10 parsecs (32.6
light years).
Note that the distance of the Pleiades is 127 parsecs. So to convert
the Apparent Magnitudes of the Pleiades to Absolute Magnitudes we must
use the conversion formula:
Absolute Magnitudes = Apparent Magnitude, V, - 5 log (distance in
parsecs/10). So at 127 parsecs, 5 log (127/10) equals 5.52. So we will
subtract 5.52 from all the V values.
"B" stands for the visual magnitude of a star looking through a blue
filter. Taking the difference between the unfiltered visual magnitude of
a star and its visual magnitude through a blue filter gives us a measure
of the star's color temperature, in this case a value called Color
Magnitude (B-V).
Complete the following table filling in the missing entries for
V - 5.52 and B-V.
V B V - 5.52 B-V
-----------------------------------------------------
2.87 2.78 Alcyone -2.65 -0.09
3.64 3.56 Atlas -1.88 -0.08
3.71 3.60 Electra -1.81 -0.11
3.88 3.81 Maia
4.18 4.12 Merope
4.31 4.20 Taygeta
5.09 5.01 Pleione
5.45 5.38 Celaeno
5.76 5.71 Asterope
6.29 6.31 etc.
6.82 6.84
6.99 7.02
7.35 7.45 1.83 +0.10
7.66 7.87
7.85 8.05
8.12 8.34
8.27 8.63
8.37 8.67
8.69 9.15
9.25 9.80
9.45 9.97
9.88 10.42
10.13 10.75
10.48 11.12 4.96 0.64
10.83 11.63 5.31 0.80
Problem 2:
Make a graph Absolute Magnitude (V - 5.52) versus Color
Magnitude (B-V) for the 25 brightest Pleiades from the above data.
Hint: Use the date from the two right hand columes to make you plot.
Choose Absolute Magnitude (V - 5.52) as the vertical axis and Color
Magnitude (B-V) as the horizontal axis. Label the axes "Absolute
Magnitude" and "B-V". Do NOT connect your data points.
Problem 3:
Explain why the absorption lines of an element have the same
wavelength as the emission lines of that element?
Problem 4:
Why are the Balmer lines weaker in the spectra of G stars than they
are in the spectra of A stars?
Problem 5:
How does the brightness of a giant star compare with the brightness
of a main sequence star of the same spectral class? If different, by
how much?
Problem 6:
Star A is 7 magnitudes brighter than star B. How does the apparent
brightness of Star A compare with Star B? Hint: Give a numeric
answer. You will need the mathematical definition of "magnitude".
Problem 7:
The time it would take for the Sun to consume all its hydrogen (if the
Sun's luminosity remained constant) would be about 100 billion years.
Why is this an unrealistic estimate of the lifetime of the Sun?
Problem 8:
Explain why the solar neutrino telescope (detector) in South Dakota
is located one mile beneath the Earth's surface and why it can operate
around the clock.
Problem 9:
Why is nearly all of the Sun's energy produced in the inner 1.5% of
its volume?
Problem 10:
What does the differential rotation of the Sun have to do with the
solar sunspot cycle?
Problem 11:
Using your planisphere, what constellation is along the southern
horizon at Midnight on your birthday?